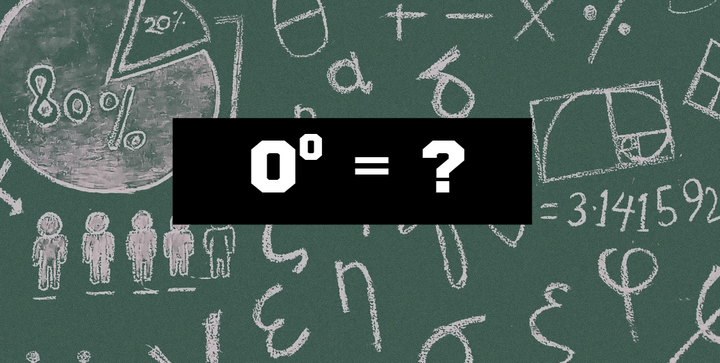Simple and Compound Interest: More Than What It Seems | Part 2

Last article, we dealt with the intricacies of simple interest. This article, we will cover the ideas and theories surrounding the basics of compound interest.

In lay man's terms, simple interest only generates interest on principal (therefore the graph for \$ over time will increase at a constant rate), whereas compound interest generates interest on interest plus principal (therefore the graph for \$ over time will increase exponentially).

The compound interest theorem

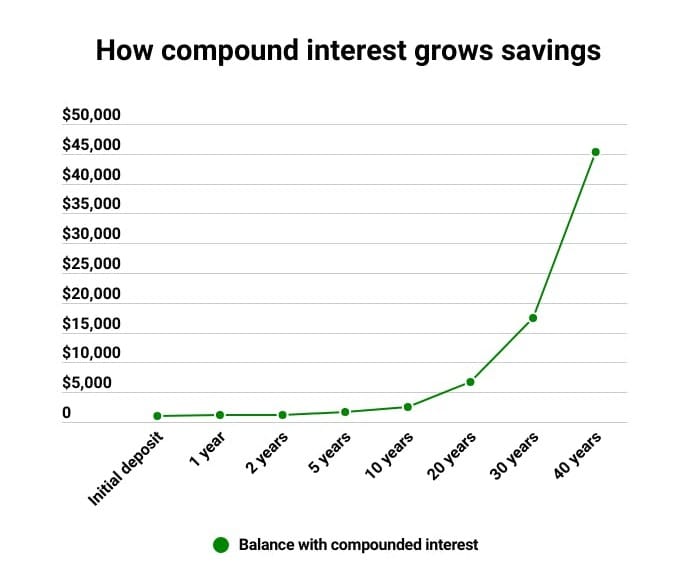
Suppose you invest RM1,000 at 10% per annum (per year), compounded annually for 5 years. After one year, you will earn 10% of RM1,000 in interest, which is RM100. Combining that interest with the principal amount gives RM1,000 +RM100 = RM1,100. At the end of the second year, this new amount earns 10% interest, that is, RM110, giving a new amount of RM1,100 + RM110 = RM1,210. If we continue doing this for 5 years, then at the end of the fifth year we have RM1,610.51. Table 2.1 shows the details:

And the line chart is as shown:

Let's derive the general formula for compound interest. Same with simple interest, we start by letting $n$ measure the total number of interest periods, of which we assume that there are $m$ times per year. The total amount we have at the end of $n$ interest periods is called the future value (or accumulated principal), and the annual interest rate is called the nominal rate. Recapping from the previous post on simple interest, we have:

1. Initial principal, $P_{0}$ : Value of investment, or present value, at year 0 ($y_{0}$).
2. Total number of interest periods, $n$: Number of times when interest is calculated across $n$ years.
3. Future value, $P_{n}$ : The future value of $P_{0}$ after the nth year.
4. Number of interest periods per year, $m$: Number of times when interest is calculated per year.
5. Nominal rate/Quoted rate/Stated rate, $i^{m}$ : The annual interest rate, expressed as a decimal.
6. Interest rate per interest period, i: $$i=\frac{i^{m}}{m}$$

We want to find a formula for the future value $P_{n}$, and we do this by looking at $n = 1$, $n = 2$, and so on, so as to hope to see a pattern. Using this notation, we can rewrite Table 1.1 using generalized formula, as shown in Table 1.2:

At the end of the first interest period where $n=1$, we received $iP_{0}$ in interest. So the future value of $P_{0}$ after the period is:
$$P_{1}=P_{0}+iP_{0}=P_{0}(1+i)$$

At the end of the second period where $n=2$, we receive $iP_{1}$ in interest, therefore the future value of $P_{0}$ after 2 interest periods is:
$$P_{2}=P_{1}+iP_{1}=P_{1}(1+i)=P_{0}(1+i)^2$$

At the end of the third period where $n=3$, we receive $iP_{2}$ in interest, therefore the future value of $P_{0}$ after 3 interest periods is:
$$P_{3}=P_{2}+iP_{2}=P_{2}(1+i)=P_{0}(1+i)^3$$

From this, we can see a pattern, which in turn suggests the following theorem,

Theorem 2.1

If we start with principal $P_{0}$, and invest it for n interest periods at a nominal rate of $i^{m}$ (expressed as a decimal) compounded m times a year, then $P_{n}$, the future value of $P_{0}$ at the end of n interest periods, is

$$P_{n}=P_{0}(1 + i)^{n}$$ where $i=\frac{i^{m}}{m}$

Example question 1: How long does it take to double your capital if you put it in an account paying compound interest at a rate of $7\frac{1}{2}$% p.a.?

Answer:

We can rephrase this question into something simpler: for what value of n does it take for $P_{0}$ to become 2$P_{0}$, if $i=0.075$? Writing this as an equation gives us:

$1.075^{n}P_{0}=2P_{0}$

Solving this gives us:

$\begin{align}1.075^{n}&=2 \\ ln(1.075^{n})&=ln(2) \\ nln(1.075)&=ln(2) \\ n&=\frac{ln(2)}{ln(1.075)} \\ n&\approx 9.58 \end{align}$

Therefore, it requires 9.58 days to double the return on an investment when interest rate is $7\frac{1}{2}$. Note that ln denotes as the natural logarithm.

If we were to make the interest rate as a variable, meaning "let $i$ be any interest rate that is above $0$", the time it takes for an investment to double, $n$, can be formulated as an equation as such:

$$n=\frac{ln(2)}{ln(1+i)}$$

If $i$ is small, $\displaystyle \lim_{i \to 0}{ln(1+i)}=i$. This is the first term of the Taylor series of $ln(1+i)$ about $i=0$. We can compute this using $f(x)=ln(1+x)$, and $a=0$ to do so:

The Taylor Series is a function in the form:

$$\sum_{n=0}^{\infty} \frac{f{n}(a)(x-a){n}}{n!}=f(a)+f'(a)(x-a)+f''\frac{(a)(x-a)^{2}}{2!}+f'''\frac{(a)(x-a)^{3}}{3!}......$$

When $f(x)=ln(1+x)$, and $a=0$,

$$n=0$: $\frac{f(0)(x-0)^{0}}{0!}=0$$

Therefore, the first term of the Taylor series of $ln(1+i)$ about $i=0$ is $i$.

Thus, we get $n\approx \frac{ln(2)}{i}$.

If, instead of $i$, we use percentage, $p$, which is $p=100i$, and we approximate $ln(2)\approx 0.693...$ by 0.72, we get $n\approx \frac{0.72}{p}$. This is the Rule of 72.

Rule of 72

The rule of 72 is an approximation on the time taken to double an investment given the fixed rate of interest. It is reasonably more accurate for lower rates (around 5% to 10%), but it is objectively more accurate if $69.3$ is used instead of $72$. The number 72, however, has many convenient factors including two, three, four, six, and nine, therefore easier for mental math.

The Rule of 72 can be applied to anything that increases exponentially, such as GDP, economic growth, or inflation; it can also indicate the long-term effect of annual fees on an investment's growth.

According to the Department of Statistics of Malaysia, from the Q1 (first quarter) of 2016 to the Q1 of 2022, Malaysia saw an average year-on-year (YoY) GDP growth of 5.6%. Applying the Rule of 72, if this percentage stays the same (or with very minimal fluctuations over the years), the economy will be expecting to double in just under 13 years:

$$\frac{ln(2)}{5.4/100}\approx 12.84$$

However, this is merely an estimate, and it has not considered any economical effects that may change the percentage, for example political instability or a pandemic such as COVID-19.

This rule was proposed by Luca Pacioli, also known as the father of accounting, in his mathematics book titled Summa de Arithmetica. However, there were no records for the derivation of this rule, as well as the reason this rule works, so many people suspect the rule predates Pacioli.

Compounding and Discounting Factor

Theorem 2.1 is valid for $n>0$, but what happens when $n<0$? This would mean that if we have accumulated $P_{0}$ over the last n interest periods at a compound rate of $i$ per interest period, what amount, $P_{-n}$ did we start with $n$ periods ago?

From Theorem 2.1, we should get

$$P_{0}=P_{-n}(1+i)^n$$, so

$$P_{-n}=P_{0}\frac{1}{(1+i)^{n}}=P_{0}(1+i)^{-n}$$

which is Theorem 2.1 with n replaced by −n. Thus, Theorem 2.1 is valid for n = 0, ±1, ±2 etc etc.

When we calculate the value of an amount of money at a future time, meaning when we calculate the future value from the present value, we talk of compounding. When we calculate the value of an amount of money at a previous time, meaning when we calculate the present value from the future value, we talk of discounting.

Suppose that we compare the original Theorem 2.1 with our new theorem (2) shown above, meaning we compare $P_{n}=P_{0}(1 + i)^{n}$ (1) with $P_{-n}=P_{0}(1+i)^{-n}$ (2).

From (1), we can say that $(1+i)^{n}$ is the compounding factor, and from (2) we can say that $(1+i)^{-n}$ is the discounting factor. It is significant, both theoretically and practically, to examine the changes in the current value when n and i changes.

Example question 2: An amount of \$5,000 (future value) is to be inherited in 4 years. What would be its current value given that the interest rate is $6\frac{3}{4}$% compounded annually? How would the answer change with these changes:

a) The interest rate goes up to 8% but the time stays the same?

b) The time extends to 6 years but the interest rate stays the same?

c) The interest rate goes up to 8% and the time is extended to 6 years?

Answer:

$$\begin{align}P_{-n}&=P_{0}(1+i)^{-n} \\ &=5000(1+0.0675)^{-4} \\ &\approx \$3850.33$ \end{align}$$

For the second part:

a)

$\begin{align}P_{-n}&=5000(1+0.08)^{-4} \\ P_{-n}&\approx \$3675.14 \end{align}$

b)

$\begin{align} P_{-n}&=5000(1+0.0675)^{-6} \\ P_{-n}&\approx \$3378.80 \end{align}$

c)

$\begin{align}P_{-n}&=5000(1+0.08)^{-6} \\ P_{-n}&\approx \$3150.85 \end{align}$

We can see the relationship between the current value, interest rate and interest period through this line graph:

We noticed that, as we increase the interest rate $i$, the discount factor and the current value decreases. The same can be said for the time, where, as we increase the interest period, the discount factor and the current value decreases. Therefore, we can make the conclusion that, although current value changes positively with the increase in discount factor, it has a negative relationship with both interest rate and interest period.

Further Development of Compound Interest Theorem

Wendy Kendrick invests \$1,000 in a certificate of deposit (CD). This will be discussed later in the article at 10% a year for five years, but when the interest is credited at the end of each year, she leaves it in her account. What amount does she have at the end of five years?

Answer:

This is a compound interest example because the interest is not withdrawn, but is added onto her principal at the start of each year. Here the principal is \$1,000 (so $P_{0}=1000$ ), the compounding is once per year (so m = 1), the interest is 10% (so $i^{1}$ = 0.1 and i=$\frac{i^{1}}{1}=0.1$), and the number of interest periods is 5 (so $n = 5$). Thus, the final amount is $P_{5} = 1000(1 + 0.1)^{5}=\$1,610.51$.

Under the same conditions, what is the future value if interest is compounded

a) Semi-annually, that is, 2 times a year

b) Quarterly, that is, 4 times a year.

c) Monthly, that is, 12 times a year.

d) Daily, that is, 365 times a year.

Answer:

a) $i^{2} = 0.10$ and $m = 2$, therefore the semi-annual interest rate, $i$, is $\frac{i^{2}}{2}=0.10/2=0.05$. This is compounded (2 times a year × 5 years) times, so the future value of $P_{0} after 5 years is:

$\begin{align} P_{10}&=P_{0}(1+0.05)^{2*5} \\ &=1000(1+0.05)^{10} \\ &\approx \$1628.89 \end{align}$

b) $i^{4} = 0.10$ and $m = 4$, therefore the quarter-annual interest rate, $i$, is $\frac{i^{4}}{4}=0.10/4=0.025$. This is compounded (4 times a year × 5 years) times, so the future value of $P_{0} after 5 years is:

$\begin{align} P_{20}&=P_{0}(1+0.025)^{4*5} \\ &=1000(1+0.025)^{20}\\ &\approx \$1638.61 \end{align}$

c) $i^{12} = 0.10$ and $m = 12$, therefore the monthly interest rate, $i$, is $\frac{i^{12}}{12}= \frac{0.1}{12}$(as exact number). This is compounded (12 times a year × 5 years) times, so the future value of $P_{0} after 5 years is:

$P_{60}=P_{0}(1+\frac{0.1}{12})^{12*5}=1000(1+\frac{0.1}{12})^{60}\approx \$1645.31 $

d) $i^{365} = 0.10$ and $m = 365$, therefore the daily interest rate, $i$, is $\frac{i^{365}}{365}= \frac{0.1}{365}$(as exact number). This is compounded (365 times a year × 5 years) times, so the future value of $P_{0} after 5 years is:

$P_{1825}=P_{0}(1+\frac{0.1}{365})^{365*5}=1000(1+\frac{0.1}{365})^{1825}\approx \$1648.61 $

This leads to the following conclusion:

If $P_{0}$ is compounded m times a year at a nominal rate of $i^{m}$, then the future value $P_{n}$ after N years is:

$$P_{mN}=P_{0}(1+\frac{i^{m}}{m})^{mN}$$

If we compound continuously, meaning the number of interest periods per year varies with no bounds, that is $m\rightarrow \infty$, but the nominal rate $i^{m}$ stays the same for varying $m$, then, because $\displaystyle \lim_{m \to \infty} (1+\frac{x}{m})^m=e^x$ for all $x$, the future value of $P_{0}$, denoted as $P_{\infty}$, after $N$ years at a nominal rate of $i^{\infty}$, is

$$P_{\infty}=P_{0}e^{i^{\infty}N}$$

If we compound RM1000 continuously for 5 years at 10%, we will have $P_{\infty}=1000e^{0.1*5}\approx RM1648.72$. If we were to tabulate this, we would get:

From this table, and from our intuition, it appears that if the nominal rate $i^m$ is the same for all $m$, then the more frequently the compounding, the greater the future value. We justify this as follows:

Theorem 2.2

If $i^m$ is positive and independent of m, and that $m\geq 1$, then the function $f(m)=(1+\frac{1^{m}}{m})^{m}$ is an increasing function, which also means that

$$(1+\frac{1^{m-1}}{m-1})^{m-1} < (1+\frac{1^{m}}{m})^{m}$$

where the inequality is bounded above by $e^{i^{m}}$, and

$$\displaystyle \lim_{m \to \infty} (1+\frac{1^{m}}{m})^{m}=e^{i^{m}}$$

We can prove this using the Arithmetic-Geometric Mean Inequality.

Arithmetic-Geometric Mean (AM-GM) Inequality:

The geometric mean cannot exceed the arithmetic mean, and they will be equal if and only if all the chosen numbers are equal. That is,

$$\frac{a_{1}+a_{2}....+a_{n}}{n} \geq \sqrt[n]{a_{1}a_{2}...a_{n}} $$

with equality only being valid when $a_{1}=a_{2}=....=a_{n}$

More precisely, the AM-GM Inequality can be written as:

$\frac{\sum_{i=1}^{n}a_{i}}{n}\geq \sqrt[n]{\prod_{i=1}^{n}a_i}$

If we select $a_{1}=1$, and $a_{2}=a_{3}...=a_{m}=1+ \frac{i^{m-1}}{m-1}$, then we get:

$\begin{align} (1(1+ \frac{i^{m-1}}{m-1})^{m-1})^{\sqrt{1}{m}} &< \frac{1+(m+1)(1+ \frac{i^{m-1}}{m-1})}{m} \\ &< \frac{m+i^{m-1}}{m}=1+\frac{i^{m-1}}{m} \end{align}$

But $i^{m-1}=i^{m}$, so

$(1+\frac{1^{m-1}}{m-1})^{m-1} < (1+\frac{1^{m}}{m})^{m}$

Because $\displaystyle \lim_{m \to \infty} (1+\frac{1^{m}}{m})^{m}=e^{i^{m}}$, the sequence is bounded above $e^{i^{m}}$.

Certificate of Deposit and Fixed Deposit

A common form of investing is through a savings product called a Certificate of Deposit (CD); CD is an application of compound interest. CDs are, in summary, a product offered by financial institutions that provides interest rate premium in exchange for the customer to leave a lump sum of deposit untouched for a specified amount of time or terms. This is more common in the US than it is in Malaysia. The most common amount of terms that are offered are usually 6 months, 1 year, 2 years or 5 years. The term will end on the maturity date, which is when the customer’s CD has fully matured which allows them to withdraw their deposits for free. Therefore, it is considered by many to be an investment with less opportunity for growth, but also comes with the perks of less volatility and a guaranteed rate of return on investment.

While the appeal of investing in a Certificate of Deposit (CD) may be tempting, it's essential to consider the potential drawbacks. One significant drawback is the risk of incurring a penalty fee or losing interest if you withdraw your funds before the maturity date. This penalty is known as an Early Withdrawal Penalty (EWP). Consequently, CDs are considered less liquid compared to other investment options like stocks or bonds. Additionally, CDs with locked rates and fixed returns can be disadvantageous if interest rates increase substantially in the future, as investors may miss out on the opportunity to invest in higher-paying CDs. It's important to note that financial institutions, such as banks and credit unions, have the authority to determine aspects of the CD agreement, including the EWP and whether the CD will be automatically reinvested if no instructions are provided at maturity.

Fixed deposits (FD) are offered by financial institutions in Malaysia as opposed to CD. Although the general structure of FD is mostly identical to CD, FD provides less fixed rate of returns compared to CD. As such, the principal of FD is usually lower than CD. In Malaysia, Perbadanan Insurans Deposit Malaysia (PIDM) offers insurance of coverage for depositors, whether it be a business or an individual. As per their regulations, “the maximum limit of coverage is RM250,000 per depositor per member bank. This includes both the principal amount of a deposit and the interest / return.” The deposits approved to be insured are:

• Savings account
• Current account
• Fixed deposits
• Foreign currency deposits
• Islamic deposits account
• Bank drafts, checks, other payment instructions or instruments made against a deposit account.

In the US, the Federal Deposit Insurance Corporation (FDIC) also provides insurance similar to that of PIDM. According to their website, "the standard insurance amount is USD250,000 per depositor, per insured bank, for each account ownership category. The FDIC provides separate coverage for deposits held in different account ownership categories. Depositors may qualify for coverage over USD250,000 if they have funds in different ownership categories and all FDIC requirements are met." The deposits approved to be insured are:

• Checking accounts

• Negotiable Order of Withdrawal (NOW) accounts

• Savings accounts

• Money Market Deposit Accounts (MMDAs)

• Time deposits such as certificates of deposit (CDs)

• Cashier’s checks, money orders, and other official items issued by a bank

References

Alhabeeb, M.J. (2012). Mathematical finance. [online] Hoboken, N.J.: Wiley. Available at: http://students.aiu.edu/submissions/profiles/resources/onlineBook/Z8P8S5_Mathematical_Finance.pdf [Accessed 12 Jul. 2023].

Chen, J. (2019). Dividend reinvestment plan (DRIP). [online] Investopedia. Available at: https://www.investopedia.com/terms/d/dividendreinvestmentplan.asp [Accessed 18 Jun. 2023].

Fernando, J. (2019). Certificate of Deposit (CD). [online] Investopedia. Available at: https://www.investopedia.com/terms/c/certificateofdeposit.asp [Accessed 19 Jun. 2023].

Fernando, J. (2023). The Power of Compound Interest: Calculations and Examples. [online] Investopedia. Available at: https://www.investopedia.com/terms/c/compoundinterest.asp#toc-compound-interest-investments [Accessed 18 Jun. 2023].

Fontinelle, A. (2019). Amortization. [online] Investopedia. Available at: https://www.investopedia.com/terms/a/amortization.asp [Accessed 18 Jun. 2023].

Kenton, W. (2019). Understanding the Rule of 72. [online] Investopedia. Available at: https://www.investopedia.com/terms/r/ruleof72.asp [Accessed 11 Jul. 2023].

Lovelock, D., Mendel, M. and A. Larry Wright (2007). An Introduction to the Mathematics of Money. Springer Link. doi:https://doi.org/10.1007/978-0-387-68111-5.

Luca Pacioli (2016). Summa de Arithmetica, Geometria, Proportioni Et Proportionalita. An Original Translation of the Distinctio Nona. Tractatus IX ‘De Computis Et Scripturis’. [online] Available at: https://archive.org/details/summa-de-arithmetica-geometria-proportioni-et-proportionalita/page/n11/mode/2up [Accessed 12 Jul. 2023].

Malaysia, D. of S. (n.d.). Gross Domestic Product (GDP) | OpenDOSM. [online] open.dosm.gov.my. Available at: https://open.dosm.gov.my/gdp [Accessed 11 Jul. 2023].

Murray, T. (2022). Business Finance Lecture Notes. [online] Available at: https://timmurrayecon.com/wp-content/uploads/2022/07/Business-Finance-Lecture-Notes-June-2022.pdf [Accessed 12 Jul. 2023].

Niesen, J. (2012). MATH1510 Financial Mathematics I. [online] Available at: http://www1.maths.leeds.ac.uk/~jitse/math1510/notes-all.pdf [Accessed 19 Jun. 2023].

Perbadanan Insurans Deposit Malaysia (2022). PIDM - Coverage For DIS. [online] Pidm.gov.my. Available at: https://www.pidm.gov.my/en/how-we-protect-you/deposit-insurance-system/coverage-for-dis [Accessed 19 Jun. 2023].

Tradingeconomics.com. (2019). Malaysia Consumer Price Index. [online] Available at: https://tradingeconomics.com/malaysia/consumer-price-index-cpi [Accessed 18 Jun. 2023].

Webber, M.R. (2022). CDs vs. Stocks. [online] Investopedia. Available at: https://www.investopedia.com/cds-vs-stocks-5225343 [Accessed 19 Jun. 2023].

Weisstein, E.W. (n.d.). Taylor Series. [online] mathworld.wolfram.com. Available at: https://mathworld.wolfram.com/TaylorSeries.html [Accessed 11 Jul. 2023].